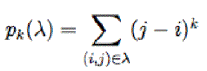An explicit formula for
the characters of the symmetric group
Page of Michel Lassalle
List of publications
Some preprints
Symmetric functions
and
Jucys-Murphy elements
Tables for
Jack polynomials :
Jack polynomials
and alpha-contents
A conjecture for
Jack polynomials
Jack polynomials
and free cumulants
This page gives some data for the normalized characters of the symmetric group.
Our results have been obtained by using a new formula expliciting these characters.
This explicit formula has been announced here and published there.
We consider the symmetric group of n letters, an irreducible representation labelled by a partition &lambda and a class of permutations labelled by a partition (&mu, 1, ..., 1).Our formula gives the normalized character
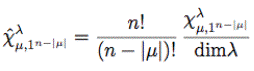
in terms of the "contents" of the partition &lambda.
Here we list these characters for any partition &mu with no part 1, such that weight(&mu) - length(&mu) < 15.
Tables giving the normalized characters for weight(&mu) - length(&mu)Tables for bigger values may be given upon request.
- from 1 to 12 (included)
(0.5 Mo)- from 13 to 14 (included)
(1.55 Mo)
Our results are in Maple format. They should be read as follows.Example :
- The partition &lambda is kept arbitrary.
The letter p_k (k > 0) stands for the k-th power sum of the contents of &lambda, i.e.
- &mu denotes a partition without any part 1.
- Each table gives first the partition &mu, then

[2]
2*p1
[3]
3*p2+3/2*n-3/2*n^2
means
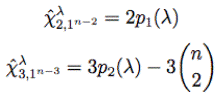
Last modified : July 18, 2009
Back to top